Thread Rating:
I am now.trying to find out with a 98.125% payout and a 3.643 variance what my 95% expected range would be on 5000 hands.
Sorry for this ridiculous post but I can not figure it out on my own.
Hello, I am trying to calculate my expected.loss range for 5000 hands of $1 video poker playing 5 credits. AKA $25,000 coin in. Here is the issue. I want to adjust the strategy to where I treat going for a.royal flush as I do any other straight flush. This reduces my win rate but greatly reduces the variance. It Appears that in 9/5 poker even with the reduced amount of royal flushes my payout would be 96.8474 if a royal was $250. However a royal is really $4,000 so that .000852 return from royal flush would be multiplied by 16 to get .013632 when we add that to the rest of the return I get a payout of 98.125% instead of the typical 98.4498% from optimized play. This knocks the variance from 19.495 to 3.643
http://wizardofodds.com/games/video-poker/analyzer/
I am now.trying to find out with a 98.125% payout and a 3.643 variance what my 95% expected range would be on 5000 hands.
Sorry for this ridiculous post but I can not figure it out on my own.
“Risk of ruin” is the chance that you go broke. For example, if your risk-of-ruin on a weekend trip to Las Vegas is 50%, it means that there’s a 50% chance you’ll lose all the gambling money you brought with you. What are the major benefits of your software for average video poker players?
'This knocks the variance from 19.495 to 3.643'Really ??? perhaps I'm misunderstanding.
Standard deviation is the square root of variance.
(3.64^0.5) * (5000^0.5) * $5 = $674
EV is .9845 - 1.00 = -1.55%.
EV for 25000 coin in is -1.55% * 25,000 = -$387.
Being within 3 standard deviations (about 96% of the time), that puts you at:
On the left (bad) side is -387 - (674*3)
On the good side, -387 + (674*3)
However, that isn't 100% perfect. Because while increasing the return (on RF) increases your return, it also increases the variance.
So same calculation, but replace the return (instead of the 98% return, plug in the 96% return). And it's somewhere in between those two.
If that makes any sense.
It isn't perfect, but yeah.
Hello, I am trying to calculate my expected.loss range for 5000 hands of $1 video poker playing 5 credits. <SNIP>
your post has nothing to do with a risk of ruin question in my opinionhow much are you trying to ruin?
your post wants to know a range of possible ending outcomes without risk of ruin
but a good question any ways
nice test
there are programs that can do this 4u
Video Poker for winners comes to mind
and Gamblers Odds (IF you can find it safely)
Sally
Being within 3 standard deviations (about 96% of the time),<snip>
aint so, in my opinionfor a normal distribution 3SD covers abouts 99.73% of the outcomes
here is what normal could look like
here is something the op is after
On the left (bad)<SNIP>
However, that isn't 100% perfect. <SNIP>
but I still could be wrong, give or take
This is easily calculated using a correct program for the task like Video Poker for Winners
then compare your answers to those you get and see (C)
in other words
ev and sd is useless for the original question
in my opinion of course
If that makes any sense.
It isn't perfect, but yeah.
no one is perfect
nice try I do say
Sally
'This knocks the variance from 19.495 to 3.643'
Really ??? perhaps I'm misunderstanding.
many do want to learn something at times, I think
please show your work
thank you
Sally
added: show your work for what makes you think you are misunderstanding, that is
Here is the issue. I want to adjust the strategy to where I treat going for a.royal flush as I do any other straight flush.
I do not get this.try again I say maybe with different words.
It Appears that in 9/5 poker <snip>
hold on now there and herewhat exactly is 9/5 poker
in video poker?
please explain the game you are wanting to play
<snip> <snip>
Sorry for this ridiculous post but I can not figure it out on my own.
computers do help
or even simulations that can be done online too
http://www.beatingbonuses.com/simulator_java.htm
(make sure they are large enough, not just 5 or 10 sessions each)
now
the distribution of 5000 hands played can be calculated once it is clear
what game you are playing and exactly what the pay table is and how you play each hand
for example
5000 hands of
9/6 JOB
prob of a loss
71.962339%
9/5 JOB
prob of a loss
79.812666%
8/5 JOB
prob of a loss
84.753248%
beware the trend!
yes, using a windows pc is easy for these type of problems (lots of software available)
and a used windows pc is very inexpensive, in my opinion,
for apple users
Sally
In video poker, bankroll requirements matter. Risk of ruin is also a factor, but only if you’re an advantage player. This page explains what bankroll management is, what risk of ruin means, and what the different kinds of video poker players are. It also examines what kind of bankroll you might need based on what kind of video poker player you are.
What’s a Bankroll?
When gamblers refer to their bankrolls, they’re talking about the amount of money they have set aside to gamble with. Their bankroll is the amount of money they can afford to lose.
How you handle that bankroll is called “bankroll management.” Some aspects of bankroll management apply to all gamblers, including video poker players, while others only apply to video poker players who play with an edge against the house.
Here’s an example of a bankroll management strategy that applies to all gamblers, including video poker players of all types:
You should only gamble with money you can afford to lose. In other words, your video poker bankroll should be separate from the rest of your money. If you need that money to buy groceries or pay the rent, it shouldn’t be part of your gambling bankroll.
Some people use win goals and stop loss limits as part of their video poker bankroll management approach. These aren’t necessary, and they don’t affect your odds of winning. But the concepts can be useful.
A win goal is an amount of money that, once won, signals that it’s time to quit for that session or that gambling trip. For example, if I have a bankroll of $1000, and I set a win goal of $100, I’ll quit playing when my bankroll increases to $1100 via winnings.
A stop loss limit is the same thing, only applied to losing money. If I had a $1000 bankroll with a stop loss limit of $100, I’d quit playing when my bankroll hit $900.
Bankroll management proponents imply that this approach improves your chances of winning. They don’t, though, because quitting at an arbitrary point doesn’t matter to the probabilities. The math doesn’t care if you quit one day and start again the next. As far as the math behind the game works, you’re playing one lifelong session, regardless of how many breaks you take during that session.
What Is Risk of Ruin?
In some situations, a gambler has a mathematical edge over the casino. This edge is usually small, though, and you can’t rely on seeing this edge until you’ve spent a significant amount of time playing. The mathematical edge is a long term expectation. Anything can happen in the short run.
This is how the casinos stay in business, by the way. They rely on having a tiny edge over you, but they understand that in the short run, sometimes you’ll win. If you didn’t sometimes walk away a winner, you wouldn’t play.
Since anything can happen in the short term, you can go broke, even if the odds are in your favor. Risk of ruin measures the likelihood of that happening.
Generally, the smaller your bankroll is in relation to your average bet size, the greater your risk of ruin is. For example, someone with a $1000 bankroll who’s playing a quarter machine is betting $1.25 per hand (5 coins). That’s 800 units.
Someone with a $500 bankroll only has 400 units in that situation.
The person with the $500 bankroll clearly has a greater probability of going broke before his tiny edge kicks in.
The other factors that determine risk of ruin in video poker include the size of your edge and the volatility of the game.
Most video poker players are playing with a mathematical disadvantage to the casino. Their risk of ruin is 100%. They might walk away a winner in the short run, but they’re not risking going broke before their edge kicks in. They don’t have an edge to wait for.
Even skilled video poker players who find the best games only get an edge of 0.2% or 0.3% versus the casino. Part of this edge comes from getting a royal flush once every 40,000 hands, too. Since you’re playing 600 hands per hour, it might take 70 hours or more to see one of those hands.
Bankroll Requirements for Recreational VP Players
When it comes to deciding how big your bankroll needs to be if you’re just playing video poker for fun, the only consideration is how much fun you’re having and how long you want to play. If you’re planning to play video poker 8 hours a day 5 days in a row, you’re getting in 40 hours of play.
That’s about 24,000 hands of video poker.
If you’re playing 25 cent machines at $1.25 per hand, you’re putting $30,000 into action over those 40 hours.
But that doesn’t mean you need a bankroll of $30,000 to play for that long. You’d only need that much money if you were losing every hand.
Assuming the house has an edge of 3%, you’d need about $900 to play for that long. If you run into some bad luck, you might run out of money early, so double that and bring $1800 to play with.
But if you play a game where the house has an edge long enough, you will eventually lose all your money. That’s just how those games work.
Bankroll Requirements for Advantage Players
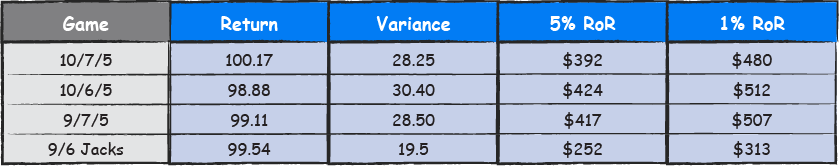
To decide on how big a bankroll you’ll need if you have an edge, you need to analyze how much of a risk of ruin you’re comfortable with. Do you want a next to 0 risk of ruin, like 1%? If so, bring a lot of money to the machines.
Video Poker Strategy
On the other hand, if you’re comfortable with a 50% risk of ruin, you can take a shot with a much smaller bankroll.
How much is that in terms of units?
You probably have a 50% risk of ruin if you bring 1000 units with you. Increase that to 7000 units, and your risk of ruin drops to about 1%.
Blackjack Risk Of Ruin
On a quarter machine, that’s the difference between bringing $1250 to the game and bringing $8750 with you.